

Next: Modelling the Global
Up: A Basis for Long-Range
Previous: Introduction
The features of any model of inhibitory cells with lateral connections
which support global competition would seem to need global connection
across the whole net. However, NRT cells have
not been observed to have connections across the whole NRT sheet, in spite
of their extensive dendrite trees (especially in the rostral part of NRT).
Thus the range over which any competition
between the NRT cells can effectively take place will
be limited. The localised properties of such competition have already
been analysed over a decade ago [17], in terms of
the even more simplified model of a single sheet with excitatory input
and a Mexican hat style of lateral coupling.
The range over which the competition can occur is
of order that of the range of connections on the sheet. This feature of the
sheet is not satisfactory for global control of the form of global guidance
as in attention and consciousness [45].
It would seem that without considerable extensions of the
lateral connections on NRT (or a small enough sheet, as might be the case
in the rat) global guidance could not arise, and attentional control would
be weak. A number of localised activities could then be supported on cortex,
in disagreement to the ability to attend only to a single object at a time.
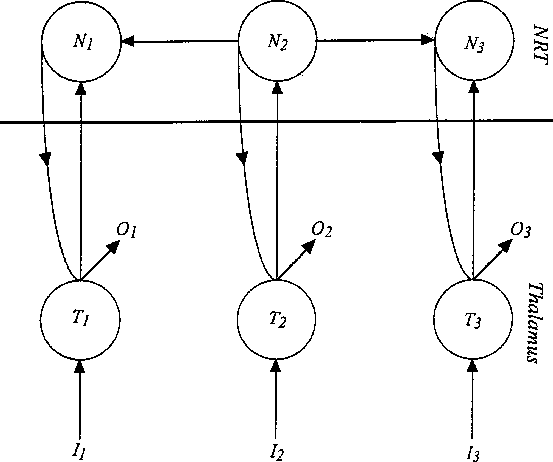
Figure 1:
The structure of the first model of the
thalamus-NRT-cortex complex, in which the cortex is dropped, and
competition occurs only between the inputs 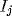 to the thalamiccompetition occurs only between the inputs
to the thalamiccompetition occurs only between the inputs 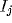 to the thalamic relay
cell
to the thalamic relay
cell 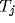 ; the strengths
; the strengths  of the outputs indicate the winners and
losers of the competition carried out between the corresponding NRT
neurons
of the outputs indicate the winners and
losers of the competition carried out between the corresponding NRT
neurons 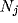 by means of their inhibitory lateral connections.
by means of their inhibitory lateral connections.
The important feature of NRT, noted at least for more advanced mammals,
above the rat, is the presence of dendro-dendritic synapses.
These latter allow the NRT to be considered as a totally connected net even
without the presence of axon collaterals of the form considered in
Figure 1.
Such dendro-dendritic synapses arise between horizontal cells in the outer
plexiform layer of the retina [15], in the form of electrical gap
junctions. They were modelled as linear resistors in the mathematical model
of the retina in Taylor [43]. On NRT, the dendro-dendritic synapses appear
to be chemical ones (as high-magnification electromicrographs show
vesicles on or on both sides of the synapses). Such synapses need to be
modelled in a non-linear fashion. In general, the dendro-dendritic synaptic
contributions to the membrane potential 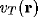 at a particular cell
at the point
at a particular cell
at the point  on the NRT sheet might be approximated as a sum of
contributions from the nearby synapsing cells, each depending on the
membrane potential differences between the cells. Thus, a typical form would
be
on the NRT sheet might be approximated as a sum of
contributions from the nearby synapsing cells, each depending on the
membrane potential differences between the cells. Thus, a typical form would
be
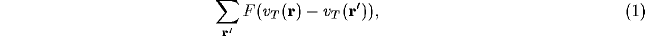
in terms of some non-linear function F. Working with only small changes
of potentials, F can be linearised, to give the contributions
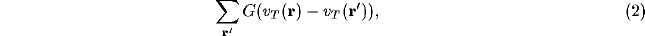
where 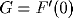 is positive in the case of inhibitory action in the
dendro-dendritic synapse. For values of
is positive in the case of inhibitory action in the
dendro-dendritic synapse. For values of 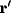 close to
close to  ,
the continuum limit can
be taken of the NRT system, and the expression 2 reduces to [43]:
,
the continuum limit can
be taken of the NRT system, and the expression 2 reduces to [43]:
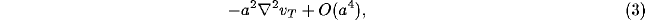
where 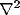 is the two-dimensional Laplacian operator in Cartesian
coordinates and a is a real constant determined by the average spacing
between the neurons of the net.
is the two-dimensional Laplacian operator in Cartesian
coordinates and a is a real constant determined by the average spacing
between the neurons of the net.
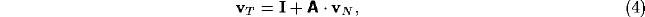
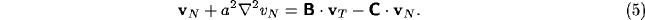
Upon neglect of the lateral connection matrix 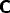 , and with
, and with 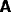 and
and
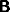 diagonal, we obtain the simpler equation
diagonal, we obtain the simpler equation
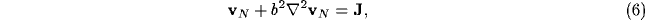
where 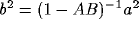 ,
,  , and we assume AB<1 to
prevent infinite gain in the thalamus-NRT feedback loop system. The expression
(6) is the basis of the simple version of the global gating model.
, and we assume AB<1 to
prevent infinite gain in the thalamus-NRT feedback loop system. The expression
(6) is the basis of the simple version of the global gating model.
What can we deduce about the dependence of the response of the NRT cells'
potentials (and hence that of the thalamic cells) from (6). We claim
that (6) instantiates a form of competition in the spatial wavelength
parameters of incoming inputs 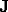 .
.
Physical systems
with this underlying description have been investigated in a number of cases:
spatially inhomogeneous superconducting states on tunnel injection of
quasi-particles [28];
a Peierls insulator under strong dynamic excitation of
electron-hole pairs or in the presence of electromagnetic radiation
[13]. These models, and related ones for growth and
dispersal in a population [38].
We may see precise forms of competition arising from the NRT modelled by
(6) by looking at these equations for inputs made of sums of plane
waves. Thus, if 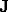 is composed of a set of separate waves of wave-numbers
is composed of a set of separate waves of wave-numbers
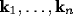 , so
, so 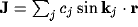 , then
for suitable coefficients
, then
for suitable coefficients 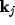 , from (6),
, from (6),
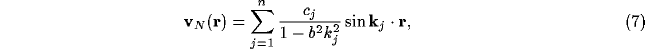
Thus, NRT activity will also display the same spatial oscillation as the input,
but now with amplification of those waves with
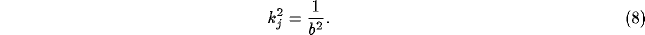
Such augmentation corresponds to a process of competition on the space of the
Fourier transform of inputs, where the Fourier transform  for
an input
for
an input 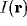 involves the global recombination
involves the global recombination
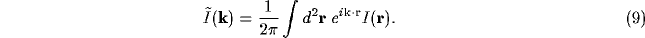
It is in this manner that we can see how NRT can exercise global control, by
singling out those components 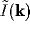 of
of 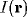 by (9) for which
(8) is true. Other values of
by (9) for which
(8) is true. Other values of 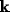 do not have such amplification. In other
words, it would appear that the NRT would oscillate spatially with wavelength
do not have such amplification. In other
words, it would appear that the NRT would oscillate spatially with wavelength
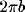 , with net strength given by the component of the input with the same
wavelength.
, with net strength given by the component of the input with the same
wavelength.
The way in which global control arises now becomes clear. Only those inputs
which have special spatial wavelength oscillations are allowed through to the
cortex, or are allowed to persist in those regions of cortex strongly connected
to the NRT: the thalamus-NRT system acts as a spatial Fourier filter. There
is evidence for this in that feature detectors occur in a regular manner across
striate cortex [27] as well as face-coding appears to
have a spatial lattice structure [23]. Other explanations
of the spatial periodicity of striate cortex feature detectors have been
proposed [16] but these are consistent
with our present proposal which may only add a further spatial instability
to that explored in those references by non-NRT processes.


Next: Modelling the Global
Up: A Basis for Long-Range
Previous: Introduction

 to the thalamiccompetition occurs only between the inputs
to the thalamiccompetition occurs only between the inputs  to the thalamic relay
cell
to the thalamic relay
cell 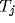 ; the strengths
; the strengths  of the outputs indicate the winners and
losers of the competition carried out between the corresponding NRT
neurons
of the outputs indicate the winners and
losers of the competition carried out between the corresponding NRT
neurons 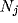 by means of their inhibitory lateral connections.
by means of their inhibitory lateral connections.
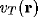 at a particular cell
at the point
at a particular cell
at the point  on the NRT sheet might be approximated as a sum of
contributions from the nearby synapsing cells, each depending on the
membrane potential differences between the cells. Thus, a typical form would
be
on the NRT sheet might be approximated as a sum of
contributions from the nearby synapsing cells, each depending on the
membrane potential differences between the cells. Thus, a typical form would
be
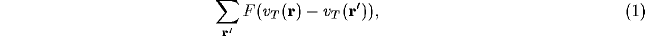
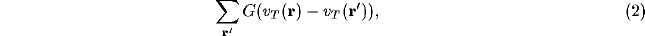
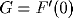 is positive in the case of inhibitory action in the
dendro-dendritic synapse. For values of
is positive in the case of inhibitory action in the
dendro-dendritic synapse. For values of 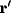 close to
close to  ,
the continuum limit can
be taken of the NRT system, and the expression
,
the continuum limit can
be taken of the NRT system, and the expression 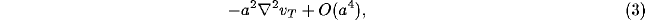
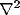 is the two-dimensional Laplacian operator in Cartesian
coordinates and a is a real constant determined by the average spacing
between the neurons of the net.
is the two-dimensional Laplacian operator in Cartesian
coordinates and a is a real constant determined by the average spacing
between the neurons of the net.
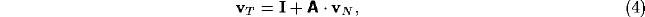
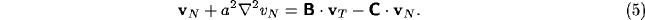
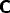 , and with
, and with 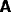 and
and
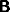 diagonal, we obtain the simpler equation
diagonal, we obtain the simpler equation
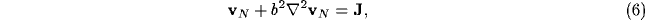
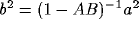 ,
,  , and we assume AB<1 to
prevent infinite gain in the thalamus-NRT feedback loop system. The expression
(
, and we assume AB<1 to
prevent infinite gain in the thalamus-NRT feedback loop system. The expression
(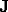 .
.
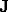 is composed of a set of separate waves of wave-numbers
is composed of a set of separate waves of wave-numbers
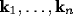 , so
, so 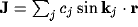 , then
for suitable coefficients
, then
for suitable coefficients 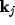 , from (
, from (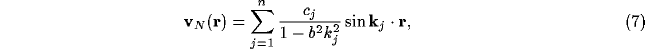
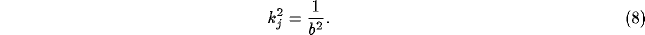
 for
an input
for
an input 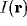 involves the global recombination
involves the global recombination
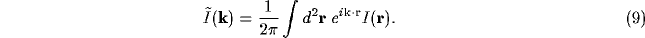
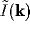 of
of 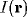 by (
by (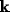 do not have such amplification. In other
words, it would appear that the NRT would oscillate spatially with wavelength
do not have such amplification. In other
words, it would appear that the NRT would oscillate spatially with wavelength
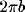 , with net strength given by the component of the input with the same
wavelength.
, with net strength given by the component of the input with the same
wavelength.